
Lyman Briggs, director emeritus of NIST, and Ossie Bluege, comptroller of the Washington Senators and, formerly, the club's third baseman and manager, at Griffith Stadium in 1959. Briggs demonstrated how to measure the spin of a pitched ball with the aid of a flat measuring tape affixed to the ball. Other Senators who assisted Briggs were pitchers Camilio Pascual and Pedro Ramos, catcher Ed Fitz Gerald, and Manager Cookie Lavagetto.
U. S. DEPARTMENT OF COMMERCE
SCIENCE AND TECHNOLOGY
Lewis L. Strauss, Secretary
Washington 25, D. C.
FOR RELEASE SUNDAY, MARCH 29, 1959 SF 59-10 (reproduced)
Scientific experiments show that the maximum curve a baseball pitcher can expect to throw is about 17 inches.
The most effective speed is about 100 feet per second, which is well within the capacity of a professional pitcher.
Speed by itself, however, has little effect. The important thing is the amount of spin. The maximum curve of 17 inches is reached at 1,800 revolutions per minute, which a professional can at least approach.
These conclusions were announced today by Dr. Lyman J. Briggs, Director Emeritus of the National Bureau of Standards, U.S. Department of Commerce, and Director of Research for the National Geographic Society. Dr. Briggs, who has been retired from NBS since 1945, conducted his experiments partly because of their value in aerodynamics and partly because he has been a baseball fan for most of his 84 years.
The serious purpose of the study is to determine the relationship of spin to deflection at different speeds. This problem has application to ballistics at very low speeds.
Dr. Briggs' research was conducted in laboratories at the National Bureau of Standards when the equipment not needed for the bureau's work, and at Griffith Stadium. There he had the cooperation of Cookie Lavagetto, manager of the Washington Senators, and several pitchers, including Pedro Ramos and Camilo Pascual. Ed Fitz Gerald was the catcher.
Two years ago a visitor to the industrial building at NBS, listening to a serious discussion of a mechanical problem by a young scientist, was startled by a loud bang a few feet in back of him. It turned out to be Dr. Briggs shooting baseballs at a paper target 60 feet away, using a large mounted air gun.
In these experiments, a baseball was rotated on a rubber tee, to give it spin, and was struck by a wooden projectile shot from the gun. The projectile drove the ball to the target. (In one wild shot, by the way, the projectile broke a window.)
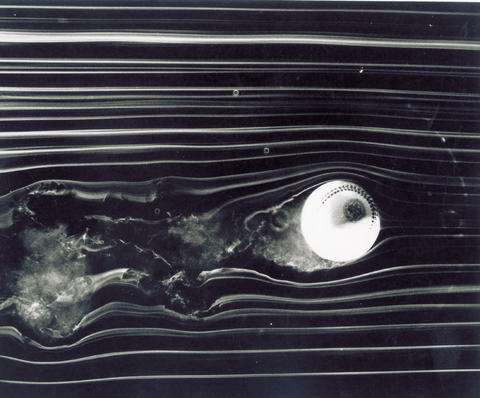
Dr. Briggs tried photographing the ball in flight from above. This gave him the speed and the curve, but it was impossible to mark the ball so as to measure the spin. Since spin was so important, he moved his experiments to the NBS wind tunnel, where speed and spin could be directly measured.
The wind tunnel experiments (described in Dr. Briggs' statement below) showed that an increase in the speed of the pitch beyond 100 feet per second reduced the curve only slightly and that the important thing was the spin.
The results of the research will be presented by Dr. Briggs in an article for a scientific publication.
Born on a farm north of Battle Creek, Mich., on May 7, 1874, Lyman J. Briggs never attended high school, but entered Michigan State College by examination at the age of 15. Four years later he was graduated second in his class. He played in the outfield on a Michigan State baseball team.
The National Bureau of Standards (of which he was Director 1933-1945) conducted various experiments with golf balls at the request of the U.S. Golf Association and with baseballs at the request of the War Department and joint committee of the American and National Baseball Leagues. In 1945 Dr. Briggs published a technical paper, "Methods for Measuring the Coefficient of Restitution and the Spin of the Ball." Coefficient of restitution refers to resiliency or bounce. He became intrigued by the effect of spin and speed on baseball curves, but put off his own research until his retirement from Government.
Dr. Briggs is an outstanding physicist. In 1939 President Roosevelt made him chairman of the original Uranium Committee to study the possibility of using atomic energy in warfare. He directed much of the early research leading to production of the atomic bomb.
Dr. Briggs' Statement
His statement on the baseball curve experiments follows:
Everyone who has played baseball or golf or tennis knows that when a ball is thrown or struck so as to make it spin, it usually curves or moves sidewise out of the vertical plane in which it started.
What makes the ball curve? To answer this question, let us imagine that the spinning ball with its rough seams creates around itself a kind of whirlpool of air, that stays with the ball when it is thrown forward into still air. But the picture is easier to follow if we imagine that the ball is not moving forward, but that the wind is blowing past it. The relative motions are the same. Then on one side of the ball, the motions of the wind and the whirlpool are in the same direction and the whirlpool is speeded up. On the opposite side of the ball, the whirlpool is moving against the wind and is slowed down. Now it is well known from experiments with water flowing through a pipe that has a constriction in it that the pressure in the constriction is actually less than in front of or behind it; the velocity is of course higher. Hence on the side of the spinning ball where the velocity of the whirlpool has been increased, the air pressure has been reduced; and on the opposite side, it has been increased. This difference in pressure tends to push the ball sidewise or to make it curve. It moves toward the side of the ball where the wind and whirlpool are traveling together.
This explanation was first given 100 years ago by a German engineer named Magnus, to account for the curved path of a cannon ball. It is known as the Magnus effect.
To what extent does the curve of a baseball depend on its spin and speed? In such measurements, it would of course be more realistic if the ball were actually thrown by a pitcher, and we could photograph its flight path with a stroboscopic camera flashing say 20 times a second. But such measurements are hard to make. I tried something like this with a ball propelled from an airgun, the camera being suspended 30 feet directly above a part of the flight path. This gave the speed and curvature when the various positions of the ball were projected in the photograph on a measuring scale on the floor below. But the images were so small that the marks put on the ball to measure the spin could not be seen. Since the spin is so important in making a baseball curve, this line of attack was given up in favor wind tunnel experiments, where the speed and spin could be directly measured.
In the wind tunnel experiments, the baseball, spinning at a known speed (revolutions per minute) about a vertical axis, was dropped to fall freely across the onrushing horizontal wind-stream, the speed of which (in feet per second) was known. The ball curved laterally across the tunnel during this fall, and its point of impact was record by a light smear of lamp black on the bottom of the ball striking a sheet of cardboard fastened to the floor of the tunnel. The time of fall across the wind-stream was 0.6 second.
As a result of many measurements it was found for spins up to 1,800 rpm and wind speeds up to 150 ft/sec that the lateral deflection (curve) of the ball was directly proportional to the spin and to the square of the wind speed, within small experimental errors.
We have now to transpose these wind tunnel measurements to conditions encountered in play. We are here concerned with the time required for the gall to move approximately 60 feet from the pitcher's rubber to the home plate. If the pitch had an average speed of 100 ft/sec, then it would take 0.6 sec for the ball to traverse this distance. But this is exactly the time required for the ball to fall across the wind-stream in the tunnel measurements. So the measured lateral deflections in the tunnel, 11.7 inches at 1,200 rpm and 17.5 inches at 1,800 rpm, represent the maximum curvature predicted for a pitched ball traveling 100 ft/sec.
For higher speeds, the wind tunnel measurements have to be reduced, because the ball is in the 60-foot zone between rubber and plate for a shorter time, and the cross-wind forces accompanying the pitch have less time to act.
The reduced measurements are given in the following table.
| Speed (ft/sec) | Spin (rpm) | Max. Curve, in 60 feet,inches |
|---|---|---|
| 75 | 1200 | 10.8 |
| 75 | 1800 | 16.7 |
| 100 | 1200 | 11.7 |
| 100 | 1800 | 17.5 |
| 125 | 1200 | 11.4 |
| 125 | 1800 | 16.5 |
| 150 | 1200 | 11.6 |
It will be seen that the speed of the pitched ball has little effect on the amount it curves. The important thing is the amount of spin.
The values are given for a ball spinning about a vertical axis. This is the most favorable position in order to obtain the maximum curve. Usually the spin axis of a pitched ball is inclined from the vertical, which reduce the curvature. If the spin were horizontal, there would be no sidewise deflection. Assuming the ball to be spinning clockwise, as seen from the right, the resulting pitch would be a drop.
These wind tunnel measurements bracketed the conditions encountered in play. According to J. G. Taylor Spink, editor of the Sporting News, the fastest pitch of record, 98.6 miles per hour (144 ft/sec) was thrown by Bob Feller of the Cleveland Indians in 1947. This was the speed across the plate, measured with an electronic device. The next fastest pitch, 94.7 mph (138 ft/sec), was made by Atley Donald of the New York Yankees in 1939.
It is of interest to compare these fast pitches with the speed reached by a baseball when dropped from a great height. This terminal velocity, about 140 per second, was measured in a vertical wind tunnel the National Advisory Committee for Aeronautics by adjusting the wind speed until the ball just floated in the upwardly-directed stream. The air resistance was then equal to the weight of the ball.
Years ago, Charles (Gabby) Street of the Washington Senators caught a ball dropped from a window of the Washington Monument. The computed terminal velocity for free fall in a vacuum from this height was 179 ft/sec; but owing to air resistance, it could not have exceeded the 140 ft/sec measurement reported by Dr. H. L. Dryden from the NACA.
The spin of a pitched ball was measured with the cooperation of the pitching staff of the Washington Ball Club. One end of a light flat tape was fastened securely to the ball. The rest of the long tape, free from twist, was laid loosely on the ground between the rubber and the plate. After the ball had been caught, the number of complete turns in the twisted tape was counted, which ranged from 15 or 16 down to 7 or 8 turns, while the ball traveled 60 feet. Assuming that the speed of the pitch was 100 ft/sec, the maximum spin was 1,600 rpm.

