Official websites use .gov
A .gov website belongs to an official government organization in the United States.
Secure .gov websites use HTTPS
A lock (
) or https:// means you’ve safely connected to the .gov website. Share sensitive information only on official, secure websites.
Ampere: The Future
Compared with the old definition, the new definition of the ampere is refreshingly straightforward. But if you’re not a measurement scientist, it might take some getting used to:
The ampere, symbol A, is the unit of electric current. Its magnitude is set by fixing the numerical value of the elementary charge to be equal to exactly 1.602176634 x 10-19 when it is expressed in the SI unit s A [ampere seconds], which is equal to C.
By quantifying the ampere in terms of the coulomb, the new definition directly ties the ampere to an exactly fixed constant of nature — the elementary electric charge — and to the second, which is also exactly defined (in terms of the frequency of energy jumps in the cesium atom).
That arrangement reflects the close relationship between the two electrical units. The coulomb is defined as the amount of charge in a current of 1 ampere that moves past a given point during one second — about 6.241509 × 1018 electrons. To determine the value of a single elementary charge, divide 1 by 6.241509×1018, which is the number of elementary charges in a coulomb. The result is around 1.602176634 x 10-19 C, which is the number that appears in the new SI definition.
Because 1 ampere is 1 coulomb per second, 1 coulomb is therefore 1 ampere-second (s A), as it is expressed in the new definition.
Despite its clarity, the new definition does not make it easy to "realize" the ampere, or convert its definition to formal reality. It requires extremely accurate counting of a staggeringly high number of individual electrons.
According to the International Committee for Weights and Measures, there are several methods for realizing the ampere in practice. One of those involves Ohm’s law.
Many labs around the world are betting on another approach called single-electron transport (SET).
This nanoscale technique transports a stream of electrons, one by one in sequential steps, from a source onto an “island” and then finally off to a drain, where the electrons are counted and stored. This is done by manipulating the electrical state of the system so that when a single electron is moved onto the island, it blocks the arrival of a second electron from the source—until the original electron is pushed along to the drain by a second electrical manipulation.
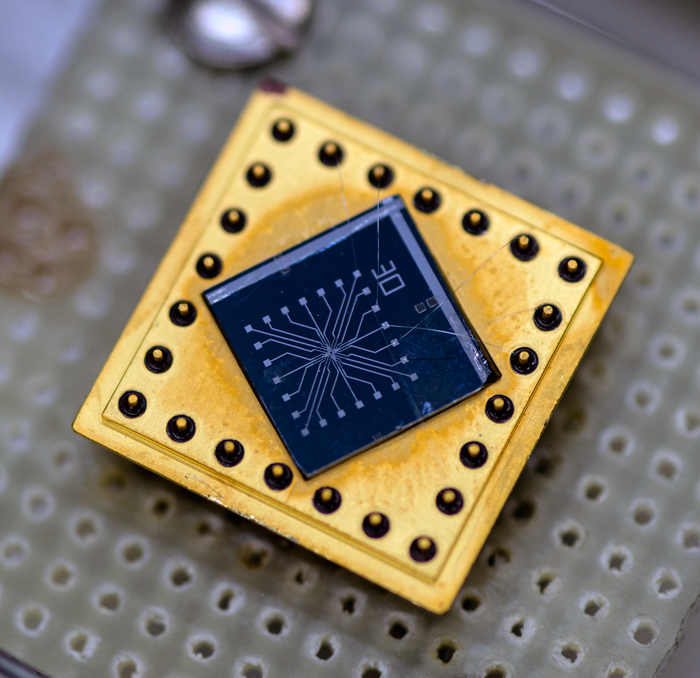
Remarkably, SET can now be performed with uncertainties as low as a few parts in a hundred million at a rate of tens of millions of electrons per second, and performance is expected to improve.
The key problem, however, is not counting individual electrons. It’s counting enough electrons. A single SET device can pump out roughly a picoampere (a millionth of a millionth of an amp, or 10-12 A) about 100 million times (108) per second. That’s still about 10,000 times too slow! Of course, realizing the ampere doesn’t absolutely require collecting the full 1018 electrons per second in 1 amp. Many experts think that achieving a current of around a microampere (millionth of an amp, 10-6 A) would be sufficient to develop a working standard. But getting there will take a lot of work.
Even 100 SET devices operating at the same time with perfect synchronization at a billion cycles per second would only produce a current in the range of nanoamps (billionths of an ampere, 10-9 A). That’s still 1,000 times less than a microamp. Researchers will also need to do accurate accounting for the uncertainties in their measurements.
But sooner or later, the quantum amp will arrive.